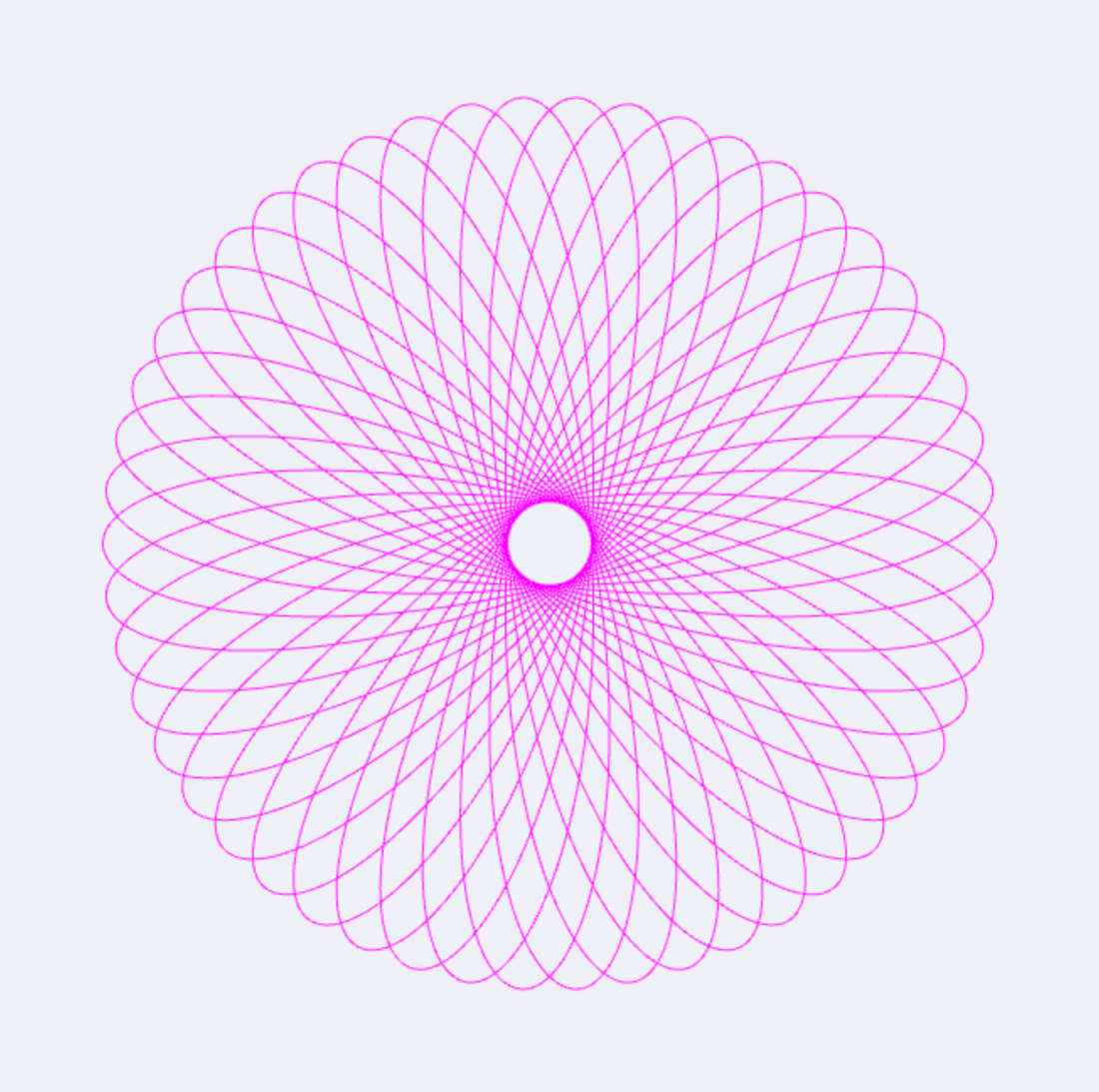
Adjust the sliders to change the values of k and l.
0.5
About the Spirograph Equations
This spirograph generator is based on parametric equations that create intricate, closed-loop patterns by tracing the path of a point attached to a smaller circle rotating inside or outside a larger fixed circle. The sliders control two key parameters in the equations: k: This parameter represents the ratio of the smaller circle's radius to the larger circle's radius. As you adjust k, you influence the relative size and shape of the spirograph's loops. l: This parameter controls the distance from the center of the smaller circle to the point being traced. Changes in l modify the complexity and curvature of the spirograph's path. The parametric equations used to generate the spirograph are:

Where 𝑡 is the parameter that controls the time progression, and 𝑅 is the base radius. By varying 𝑘 and 𝑙, you can explore a vast range of spirographic patterns, from simple circles to complex, flower-like shapes. The animation feature automatically varies these parameters over time, giving a continuously evolving visual display of spirographic art.
History of Spirographs
The spirograph holds an important place in mathematical history. The curves it produces are part of the larger family of mathematical shapes known as roulettes. By adjusting the size, rotation, and radius of the generating circles, the spirograph demonstrates the underlying principles of circular motion, ratios, and geometry, providing an accessible way to visualize complex mathematical relationships.
Early Beginnings
Mathematical Roots (Early 19th Century):
The concept behind the spirograph is closely related to the study of hypotrochoids and epitrochoids, mathematical curves traced by points linked to circles rotating inside or outside another circle. These curves had been studied by prominent mathematicians such as René Descartes and Leonhard Euler, among others. However, the practical tools to create such curves were not yet widely accessible.
Thomas Bradwardine (1300s):
Although predating the formal mathematical definition of spirographs, Bradwardine, an English cleric and scholar, explored geometric curves that are the foundations for modern spirographs. He studied spirals and curves generated through rotation, laying conceptual groundwork.
Bruno Abakanowicz (Late 1800s):
A Polish mathematician and inventor, Abakanowicz patented a device that could draw intricate geometric curves, known as an integraph. His invention was used in scientific and engineering contexts to plot curves that were difficult to draw by hand. This device helped lay the technical foundation for the spirograph mechanism.
Commercial Invention and Popularization
Denys Fisher (1965):
The spirograph became a household name when British engineer Denys Fisher invented the modern toy version of the device in 1965. Fisher, a mechanical engineer, originally created the spirograph as a tool to help design precision components for mechanical systems. However, he quickly realized the aesthetic appeal of the device’s output and adapted it as a toy.
Introduction to the Public:
Fisher exhibited the spirograph at the 1965 Nuremberg International Toy Fair, and it became a massive hit. It was soon licensed to Kenner Products, a U.S.-based toy company, which marketed the spirograph to children as an educational yet fun drawing tool. The toy was marketed with the slogan, "The magic of mathematics makes beautiful art."
1960s and 1970s:
The spirograph became a cultural phenomenon during these decades, appearing in art classrooms and homes worldwide. Its accessibility as both a learning tool for geometry and a creative toy made it extremely popular.
Modern Day:
Although the spirograph craze slowed in the late 20th century, it remains a beloved toy and teaching tool. Newer versions of the spirograph, including digital versions (like the one above) have brought the concept into the digital age, allowing users to generate intricate spirograph patterns through algorithms and animation.
Refresh the web page to change the spirograph's color.